My research interests involve permutations of these topics: point vortices, hollow vortices, compressible flows, free boundary problems, and exact solutions of the Euler equations.
Brief descriptions of some of the research projects follow, with links to corresponding publications.
If you see anything that interests you, feel free to get in touch!
For these and other projects, I collaborate with (in no particular order)
Miles H. Wheeler,
Adrian Constantin,
Darren G. Crowdy,
Mark A. Stremler,
Rhodri Nelson,
Stefan G. Llewellyn Smith and
Takashi Sakajo.
Liouville links and chains
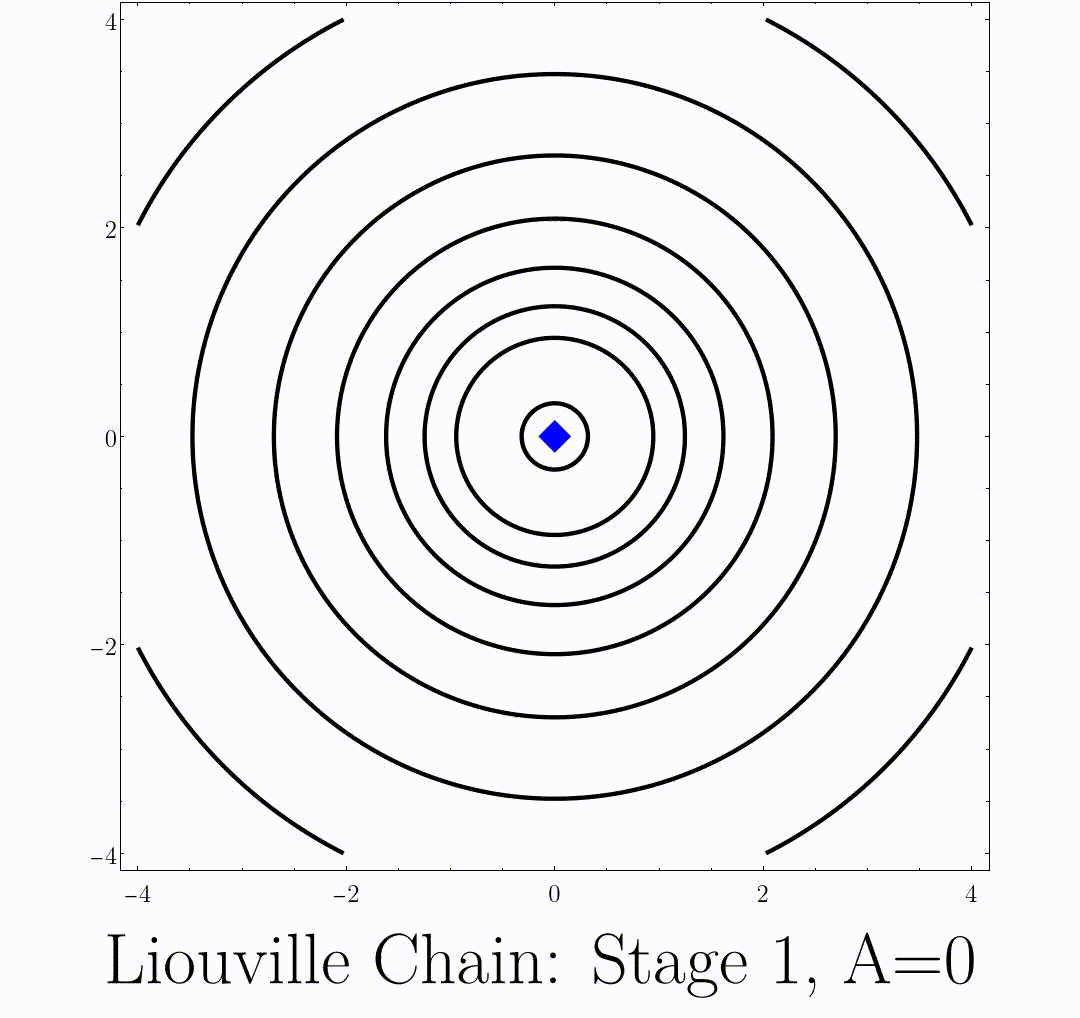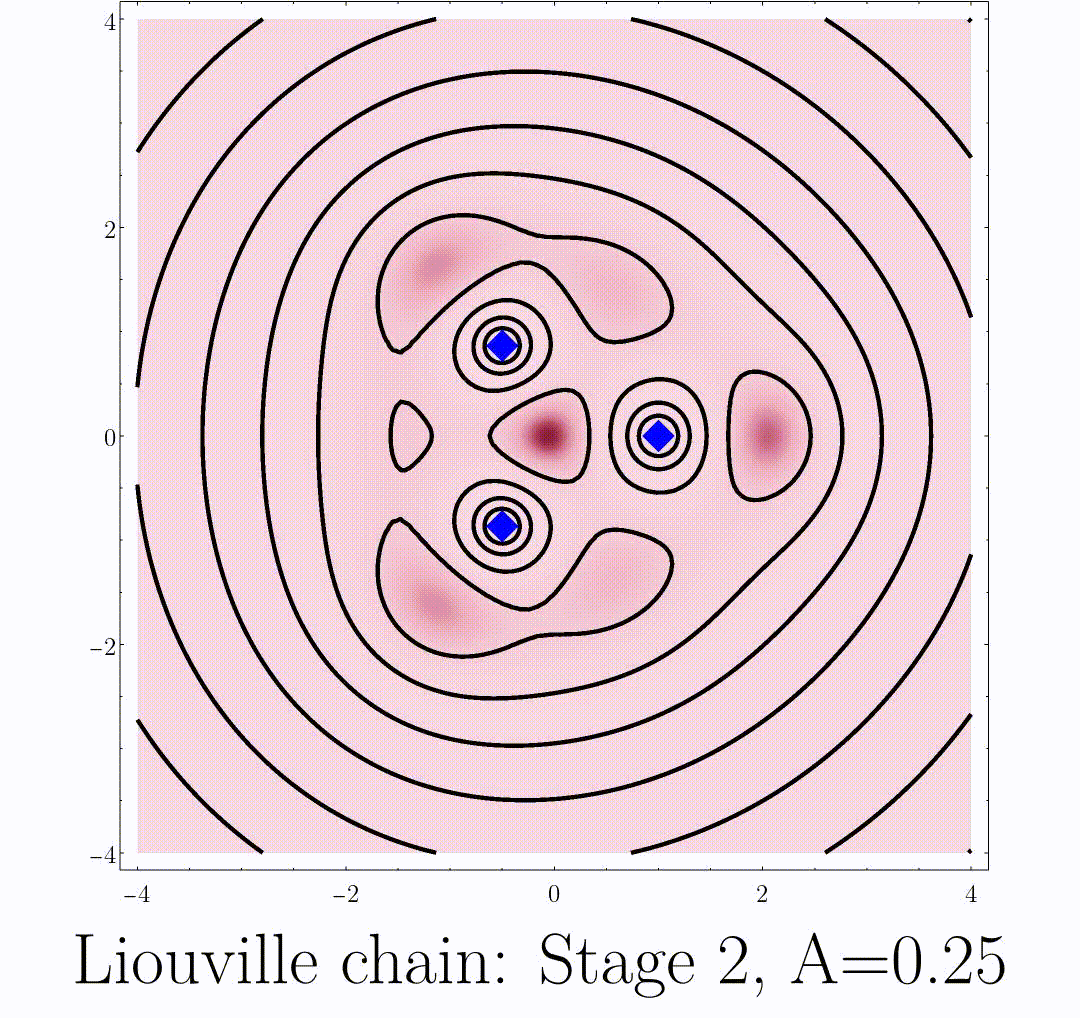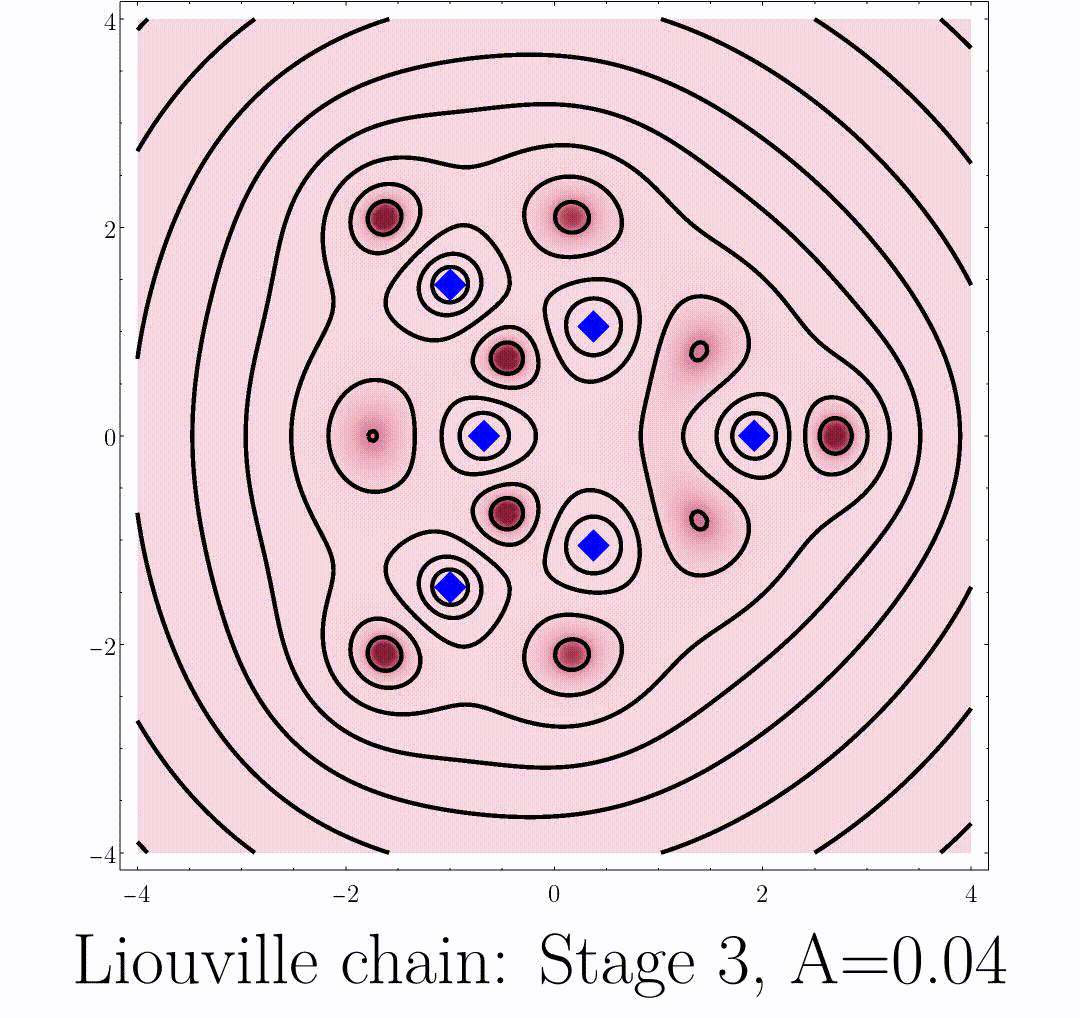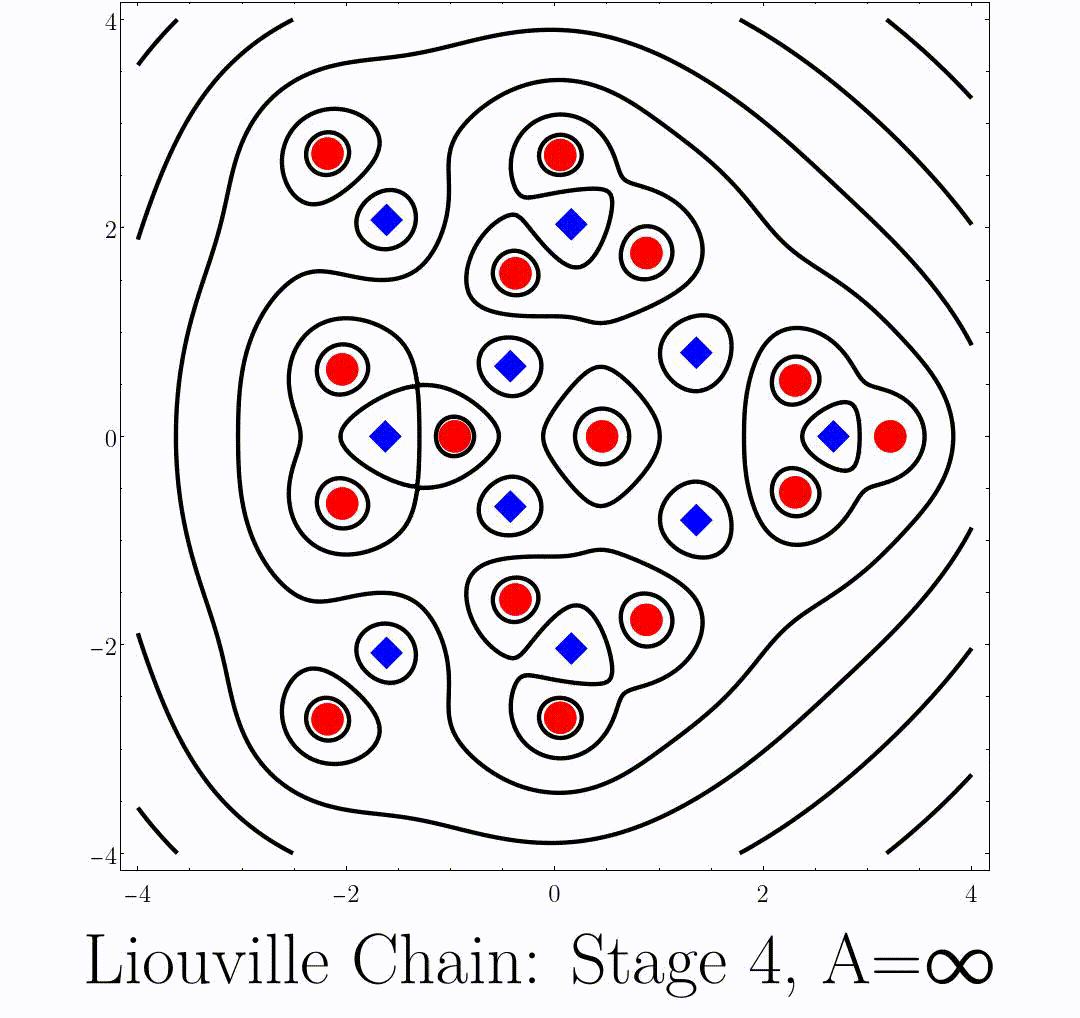
A Liouville link is a family of solutions of the steady Euler equation, continuously parametrised by some real A, whose stream function is a solution of a modified Liouville PDE.
It consists of a set of point vortices in equilibrium with a background non-constant vorticity which is non-zero everywhere and smooth except for the point vortices.
Point vortex equilibria in otherwise irrotational flow exist as limiting configurations (for A=0 and A=∞) of a Liouville link.
Several such solution families can link up to form a Liouville chain.
The point vortex circulations at the limits of a link need to be scaled to continue the chain, this is called a 'twist'.
The animation below shows an example of a Liouville chain of infinite length, given in terms of the Adler--Moser polynomials.
The vorticity heatmap shows the formation of compact regions of concentrated vorticity for finite A; in the limits these become point vortices and the background vorticity disappears.
The limits are non-uniform in space.
This work is closely related to the transformation between point vortex equilibria discussed in the section below.
References:
V. S. Krishnamurthy, M. H. Wheeler, D. G. Crowdy, and A. Constantin. Liouville chains: new hybrid vortex equilibria of the two-dimensional euler equation. J. Fluid Mech., 921:A1, 2021. [ DOI (Open Access) ]
V. S. Krishnamurthy, M. H. Wheeler, D. G. Crowdy, and A. Constantin. Steady point vortex pair in a field of Stuart-type vorticity. J. Fluid Mech., 874:R1, 2019. [ DOI | Postprint ]
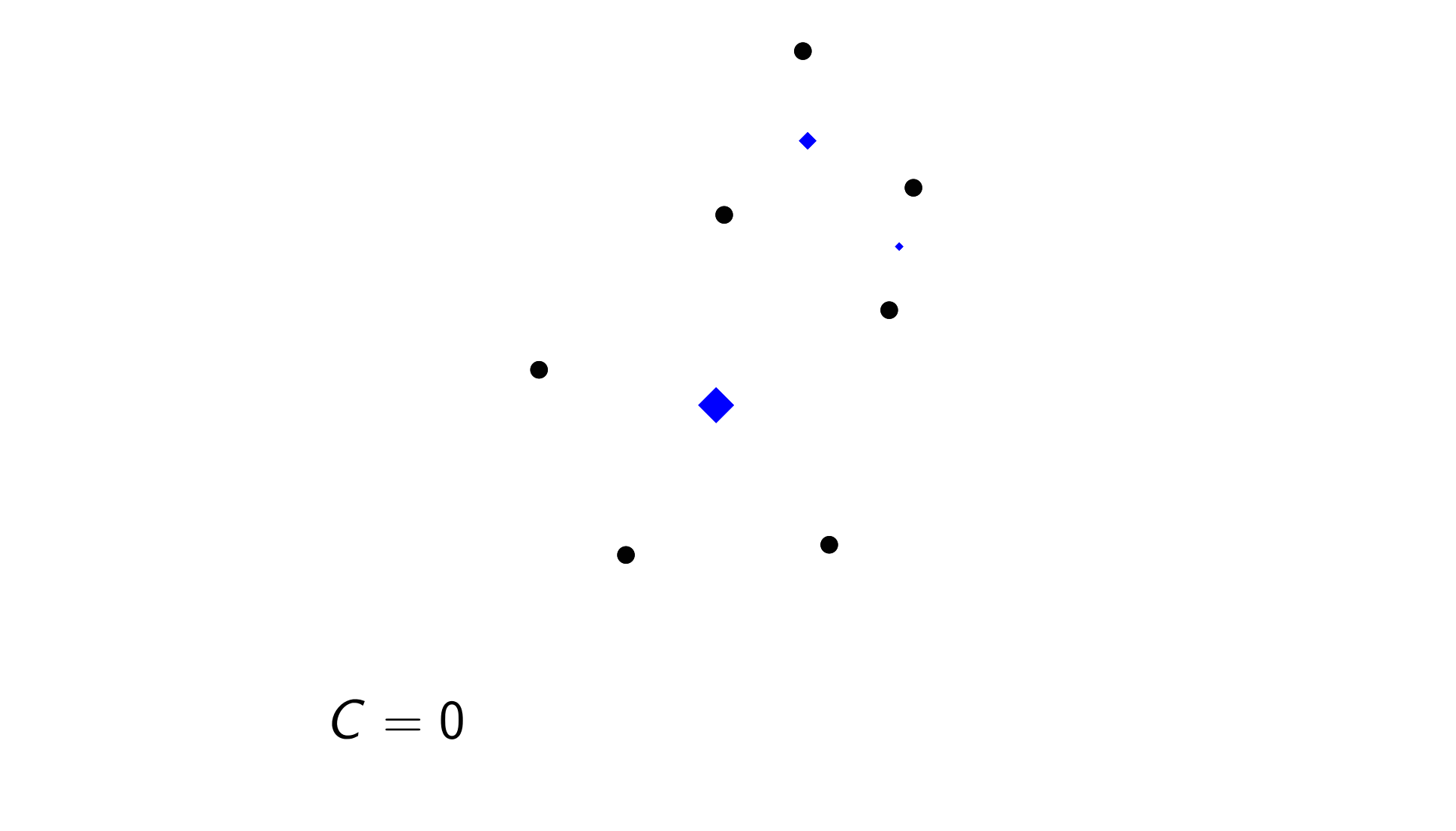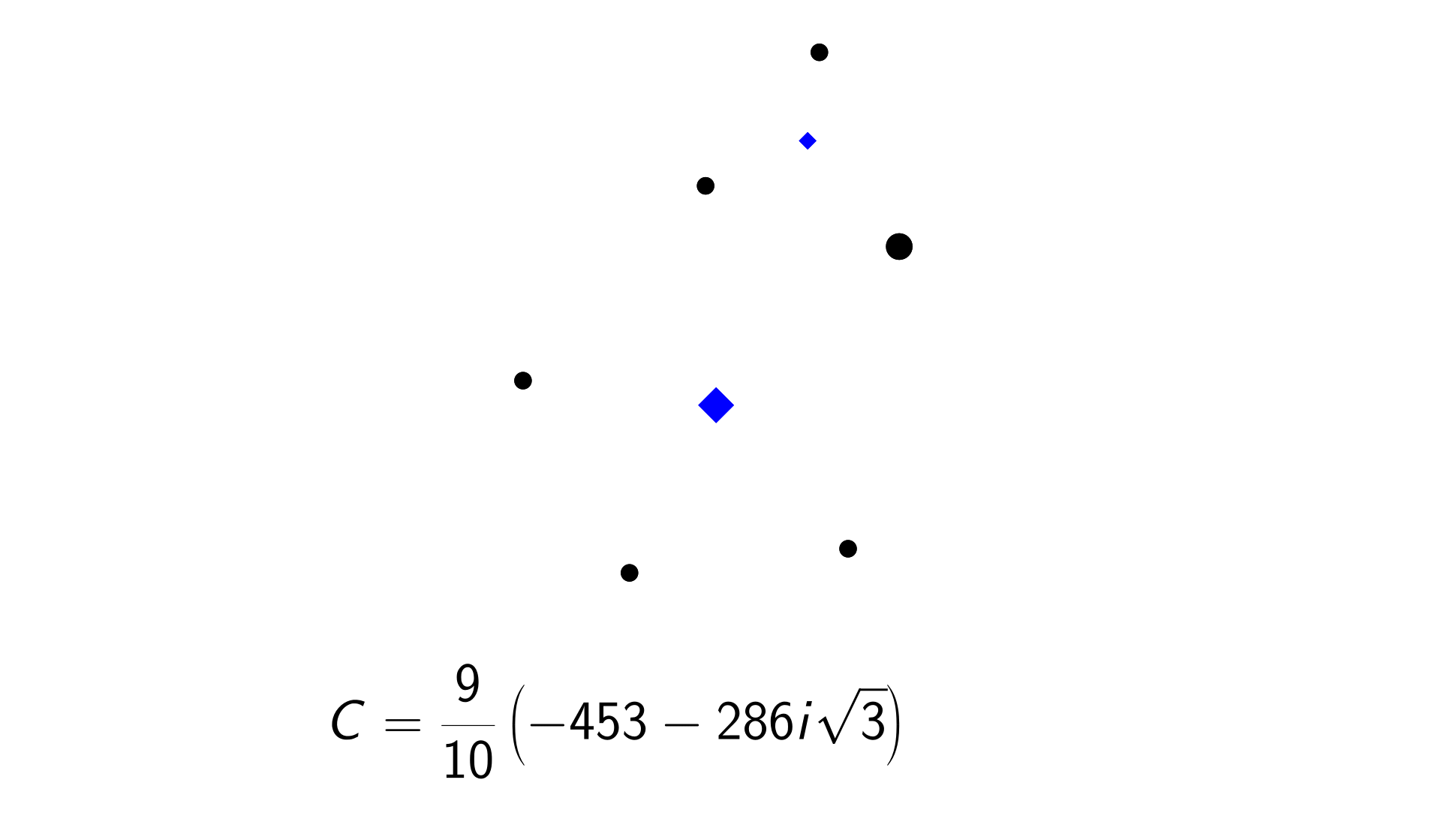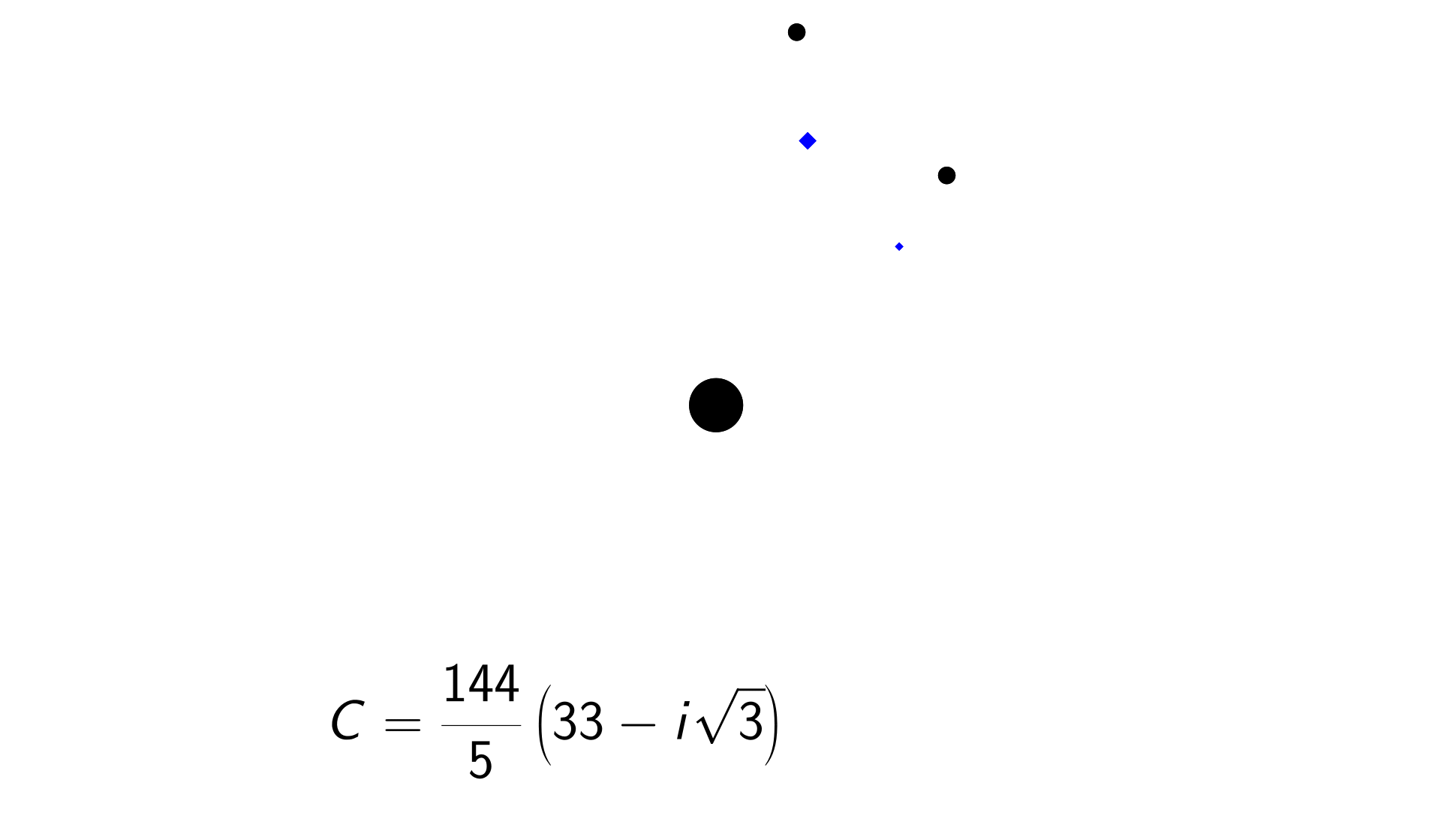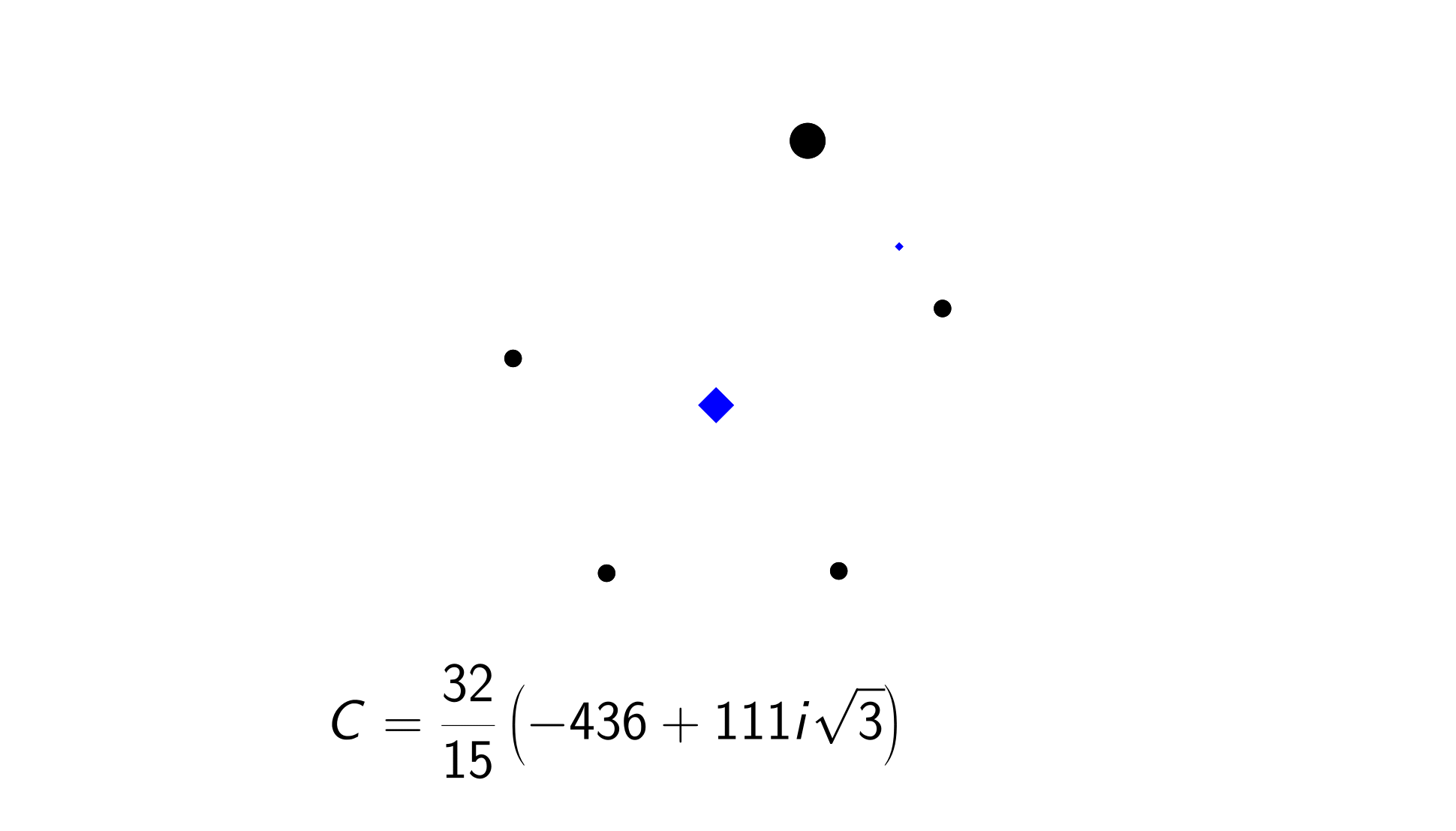
Transformation between point vortex equilibria
Point vortex equilibria are patterns of point vortices which do not move relative to one another.
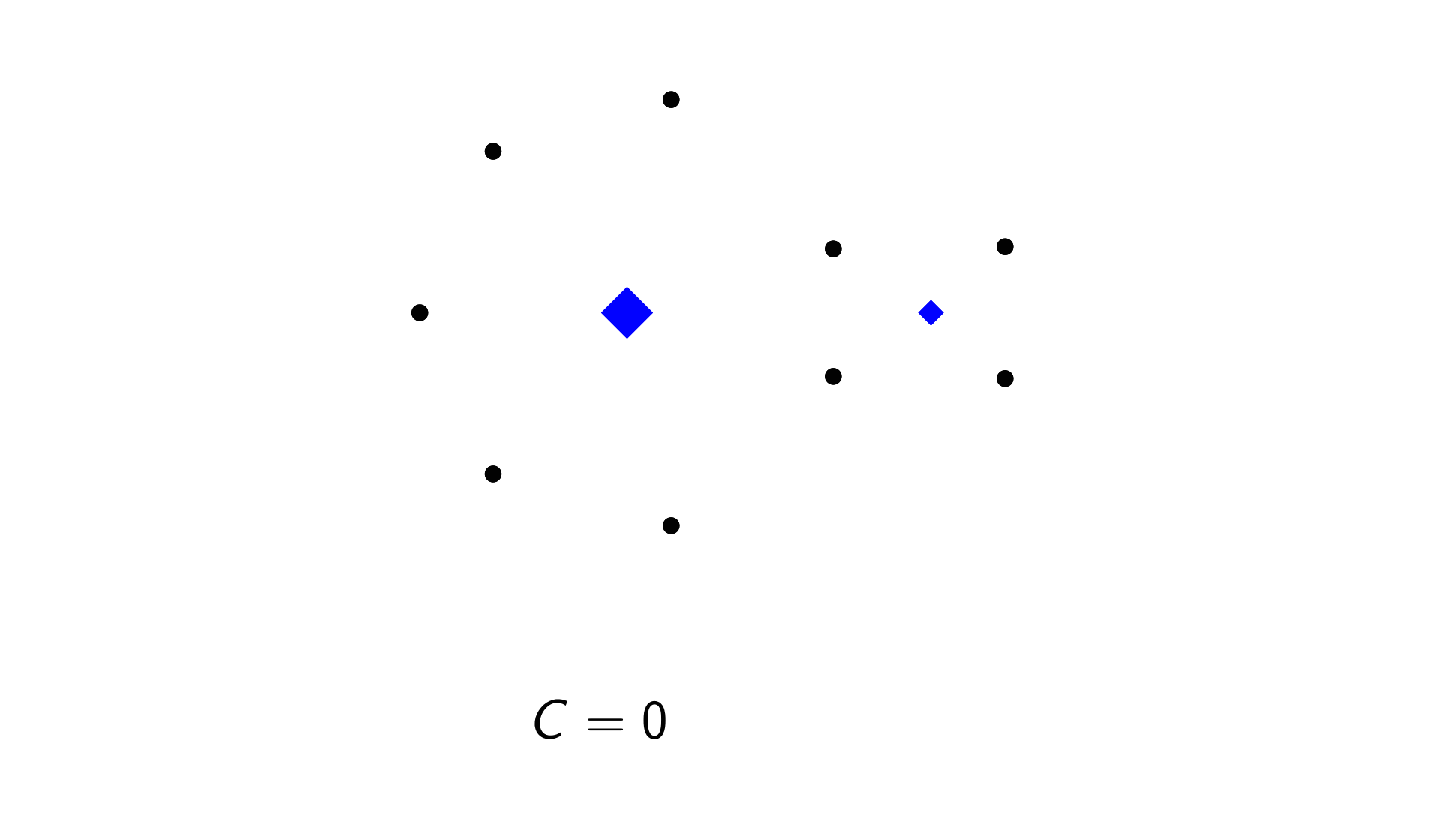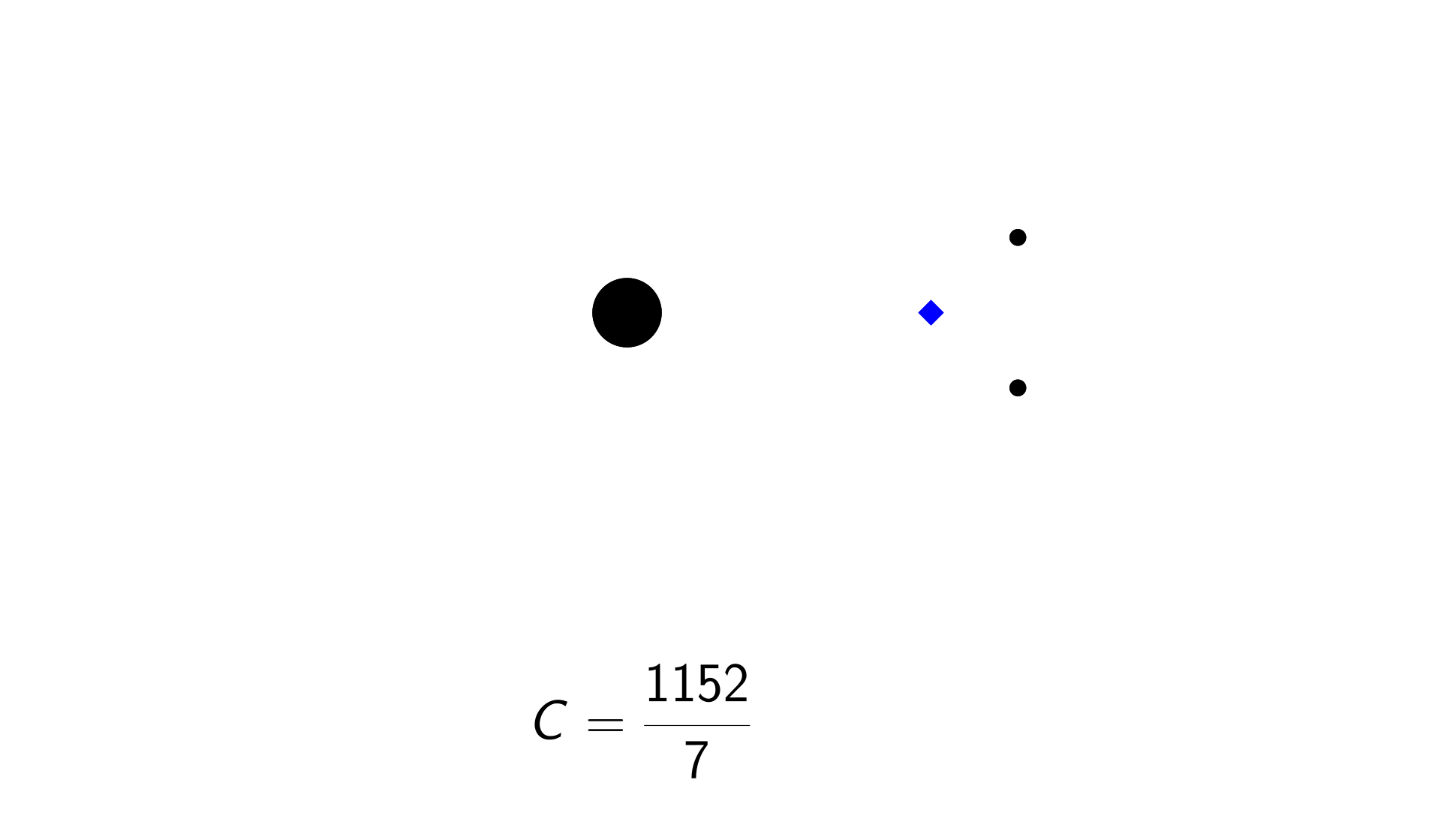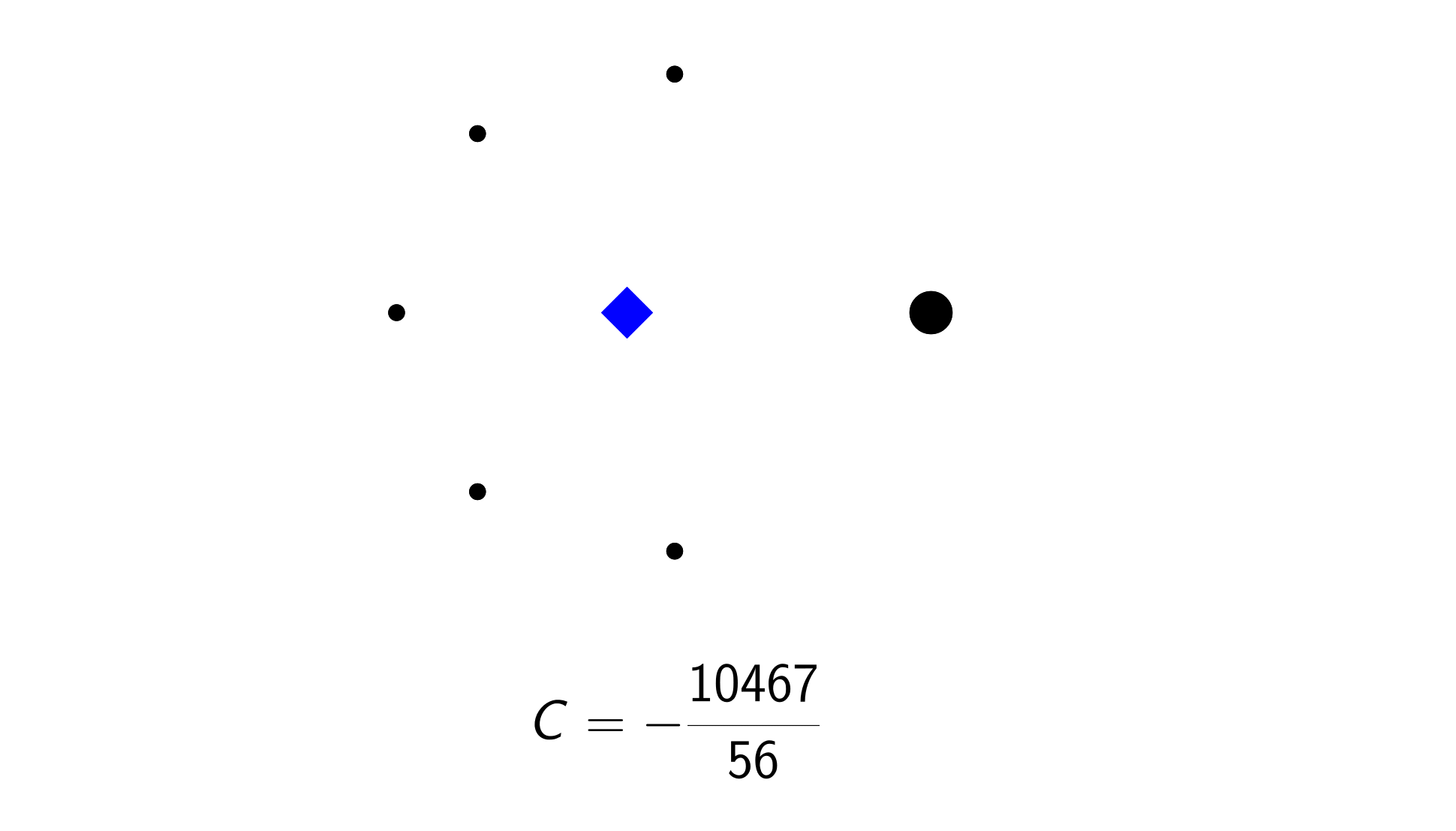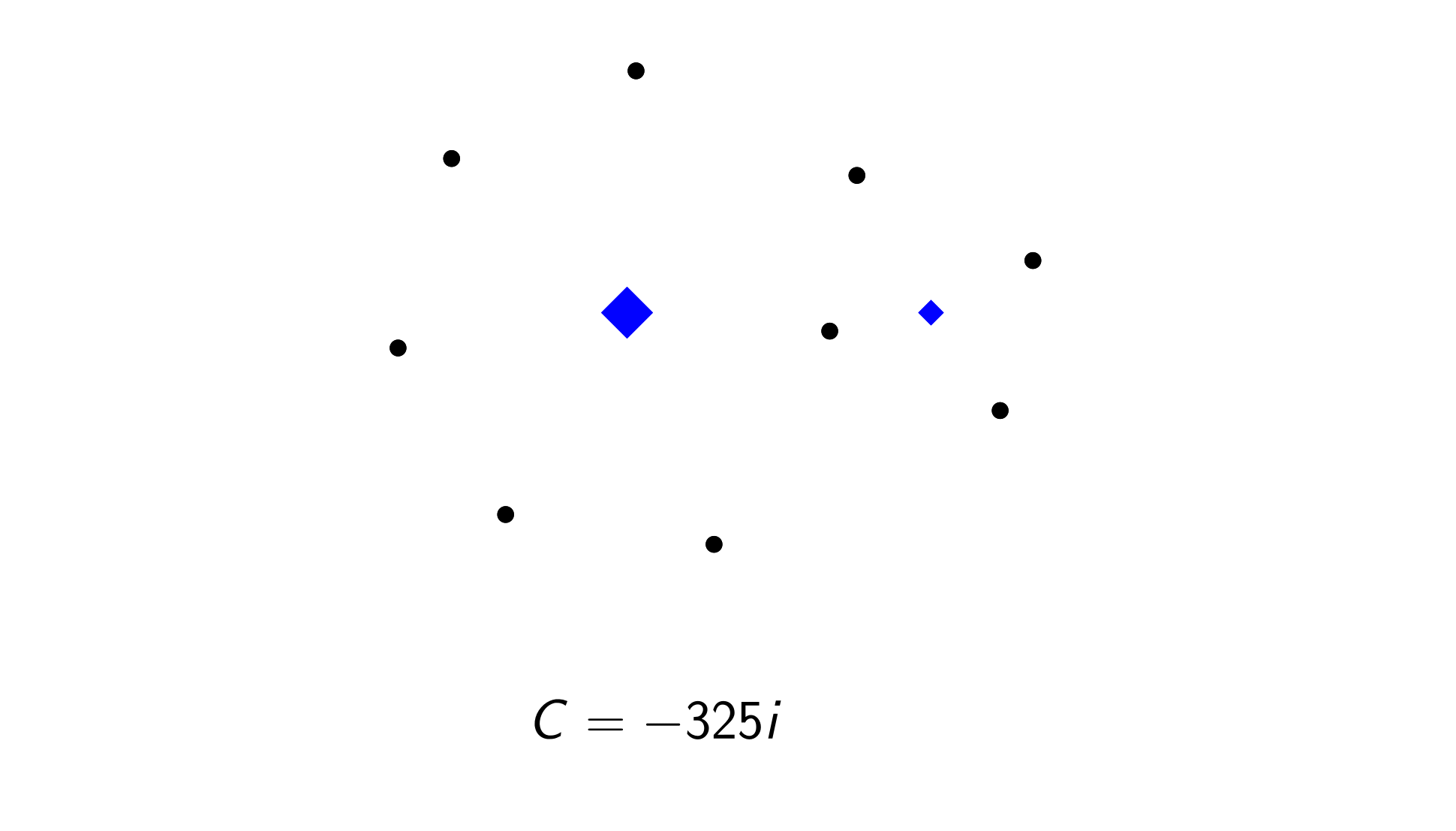
We have discovered a transformation between rational functions that takes a given stationary point vortex equilibrium and creates a new, distinct stationary point vortex equilibrium.
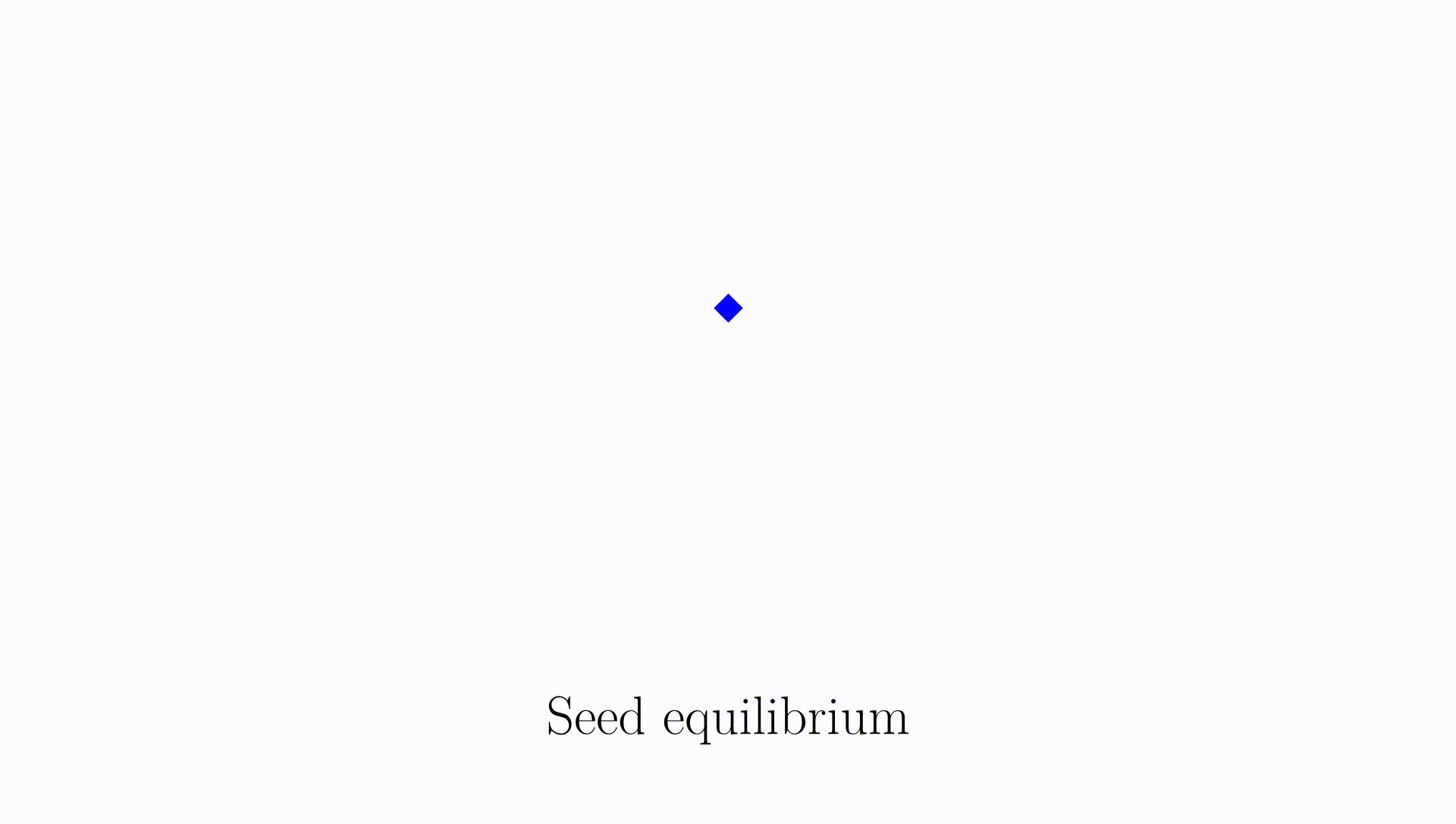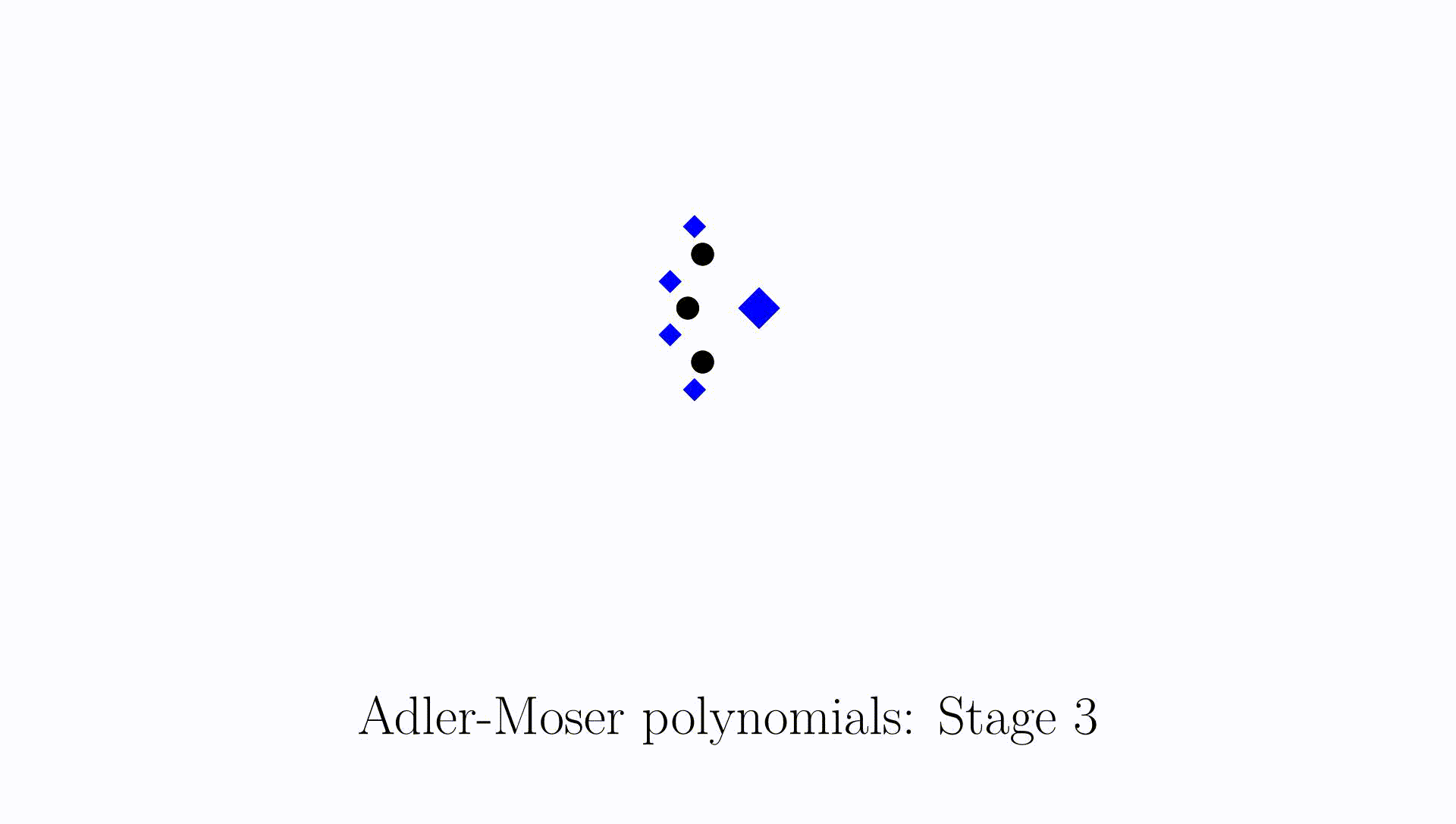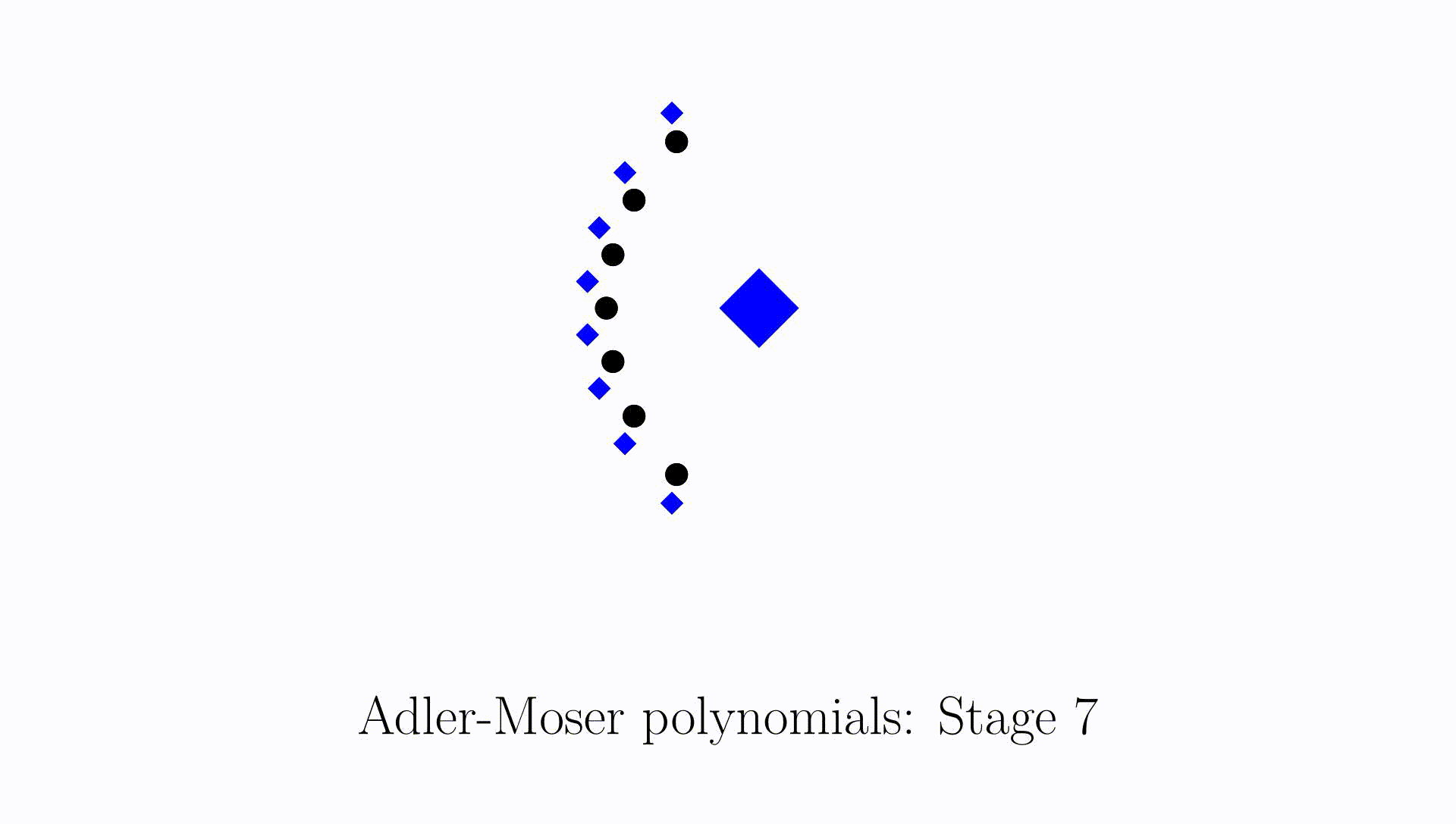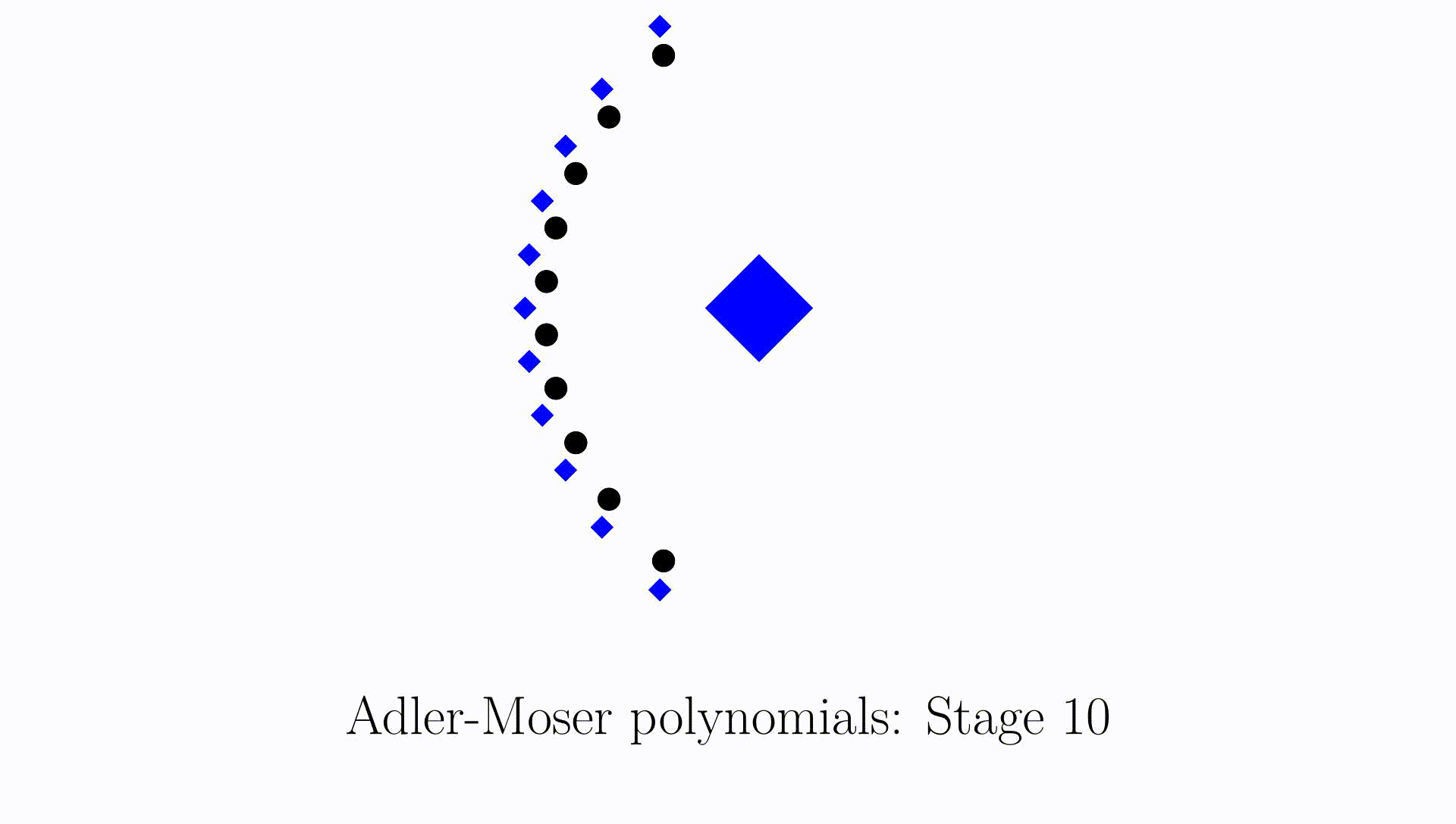
Selected examples of equilibria obtained via this transformation are shown here, as (continuous) functions of an arbitrary parameter.
Blue diamonds and black disks are positive and negative point vortices; their size gives the vortex strength.
This procedure can sometimes be iterated to produce infinite hierarchies of equilibria.
The first few stages for two such hierarchies are shown below.
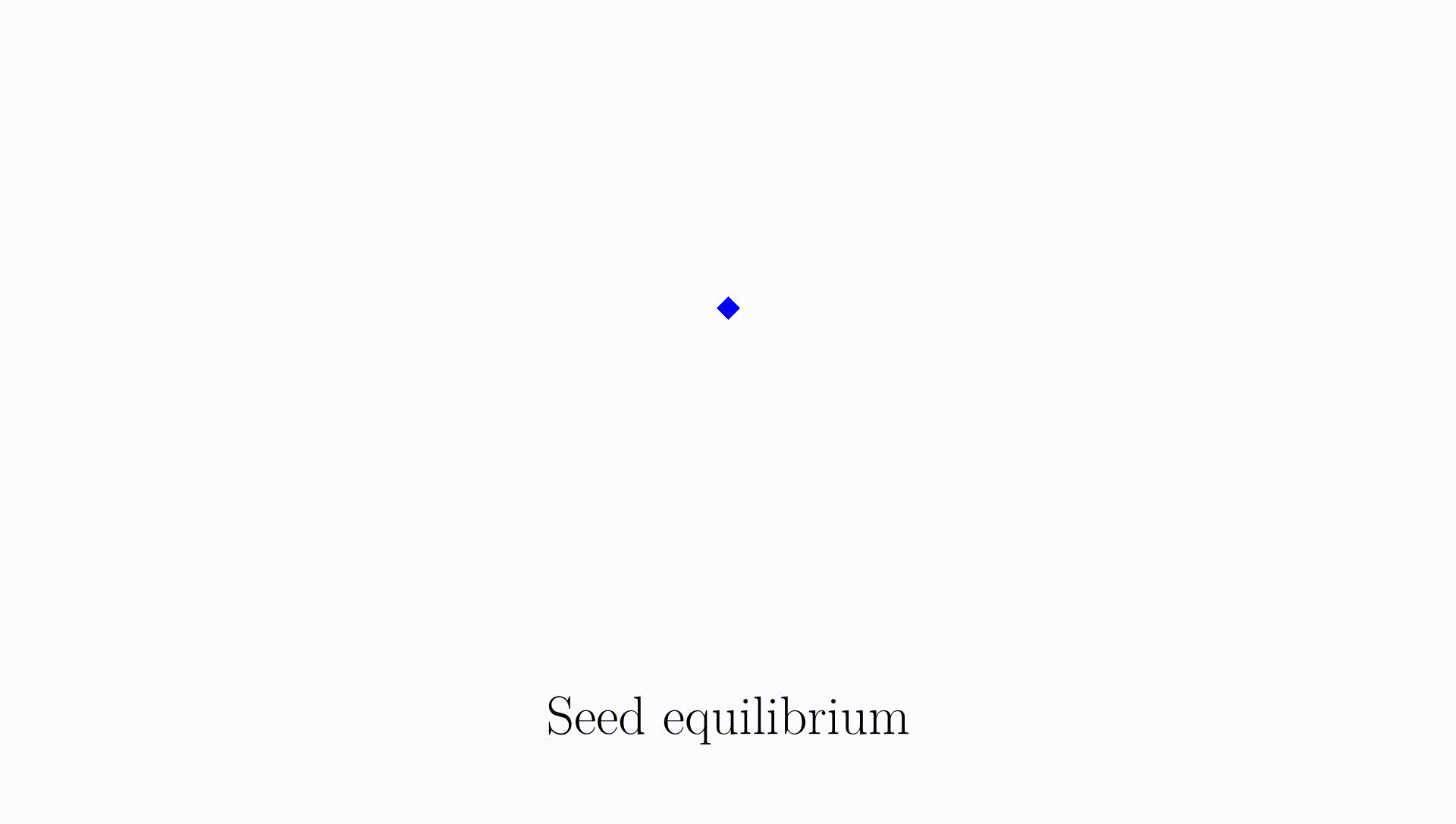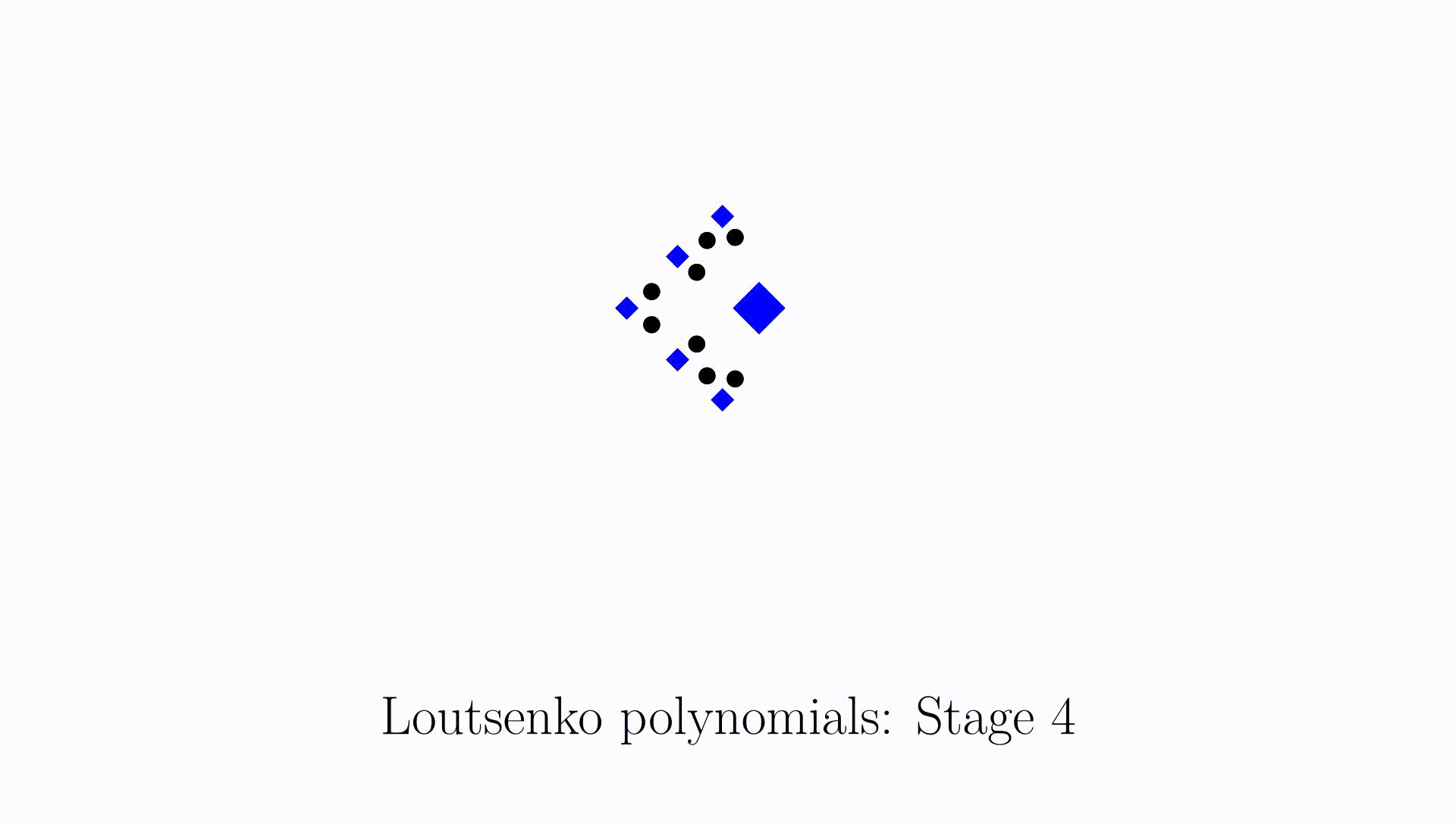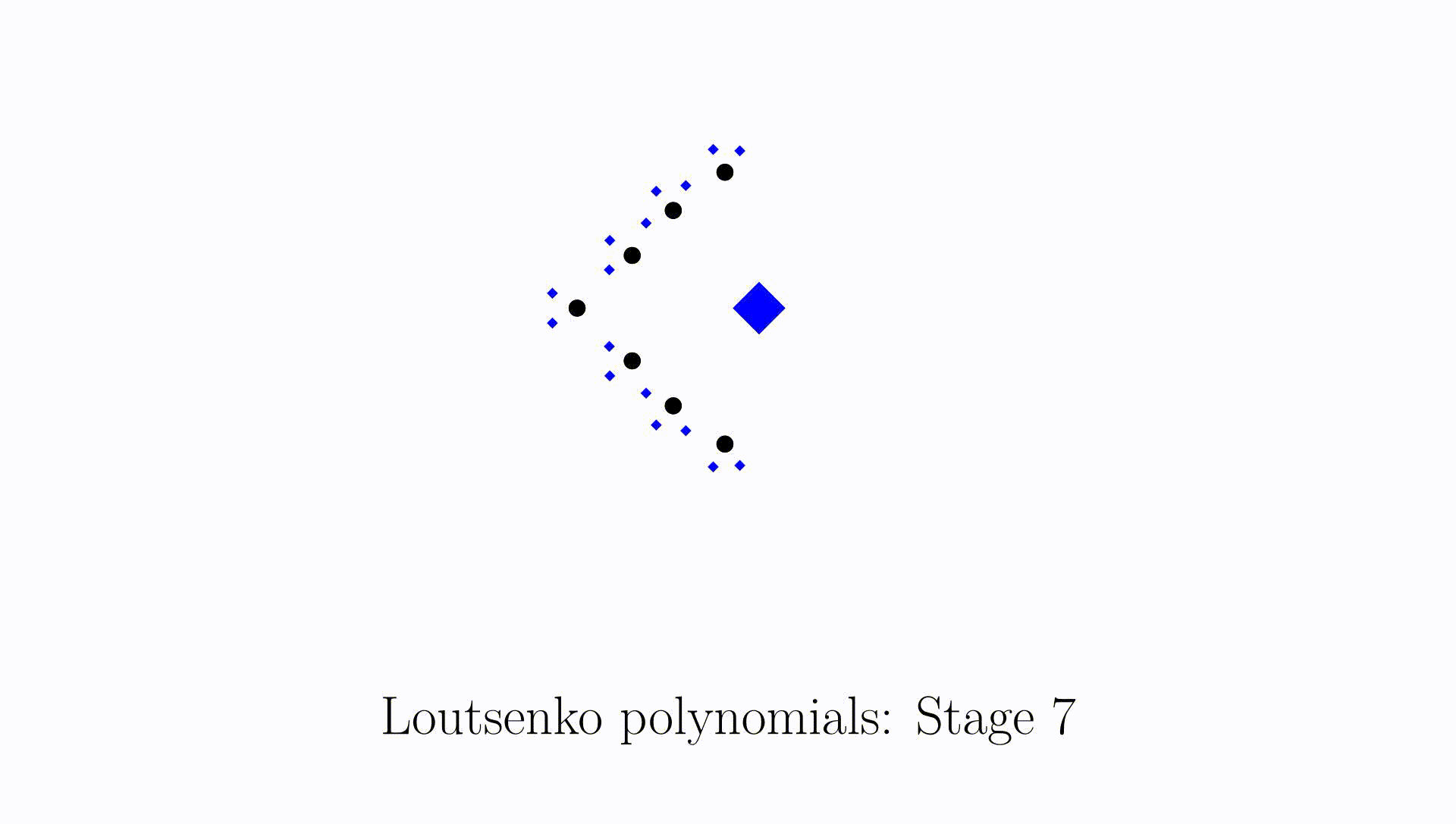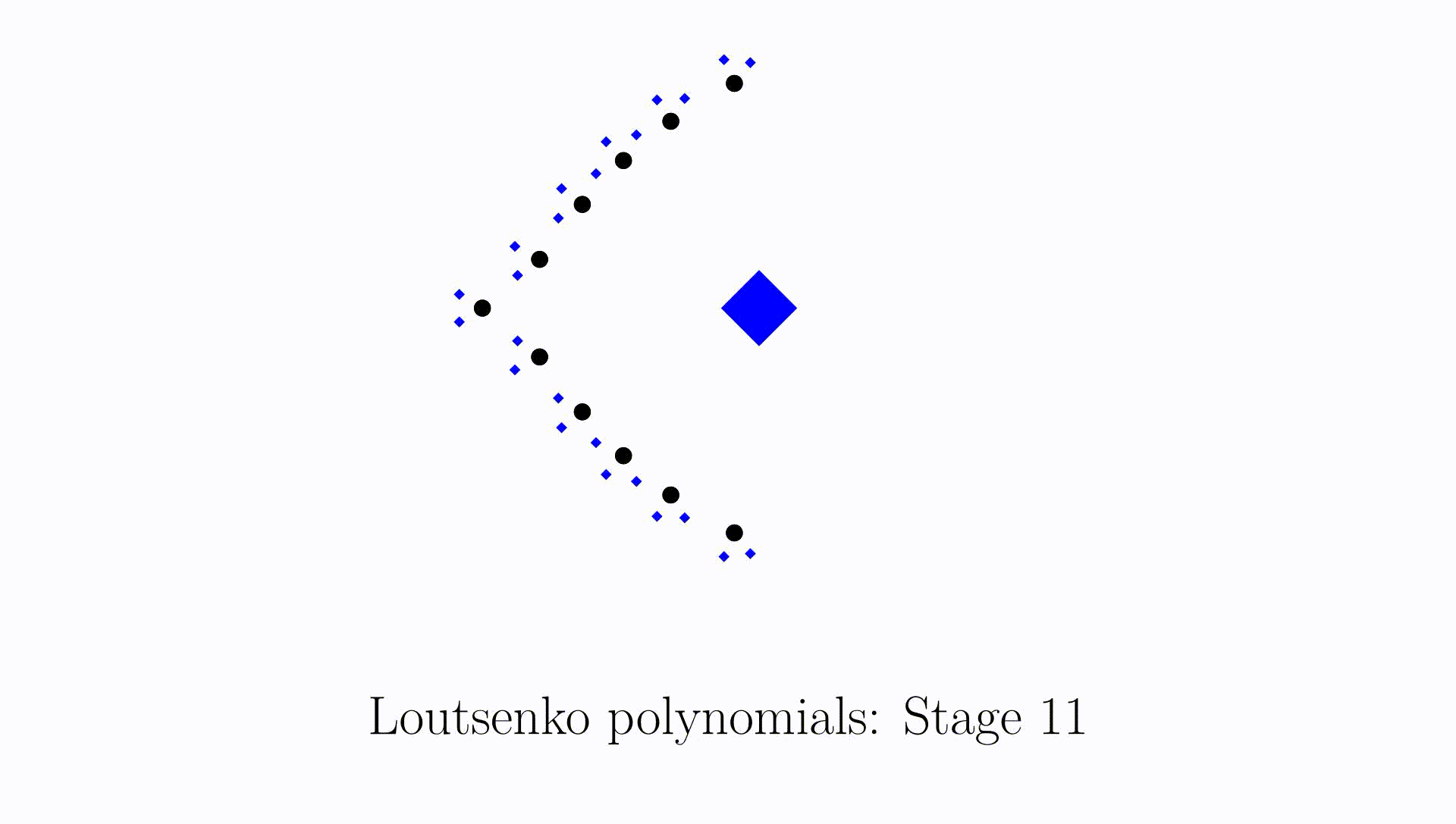
References:
V. S. Krishnamurthy, M. H. Wheeler, D. G. Crowdy, and A. Constantin. A transformation between stationary point vortex equilibria. Proc. R. Soc. A, 476(2240):20200310, 2020. [ DOI | Postprint ]
Compressible vortex dynamics
The Kármán vortex street is a widely observed feature of fluid flows. Various solutions, including the classic point vortex street model, are available for incompressible flows. For a compressible flow on the other hand, a point vortex is especially problematic due to negative densities appearing above a certain fluid speed. We can still consider a steadily translating Kármán point vortex street in a compressible flow and, after clarifying the meaning of a point vortex in equilibrium in such a flow, an exact expression for the speed of the street can be obtained. We also consider finite-area hollow vortex streets, and find that it can speed up or slow down relative to an incompressible street depending on the area of the vortices. The results depend on the geometric arrangement of the vortices, encoded in κ which is the horizontal to vertical separation of the vortices.
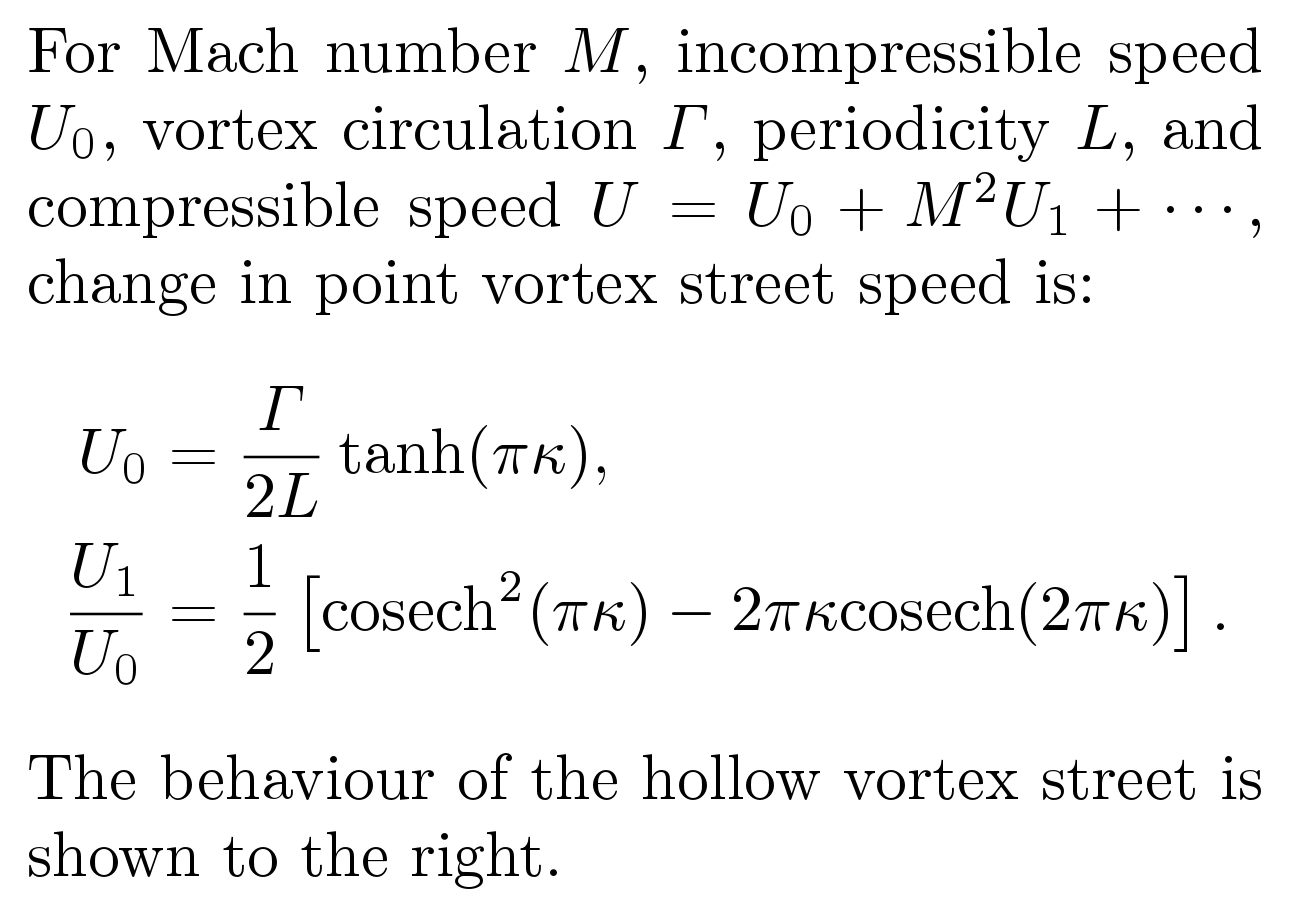
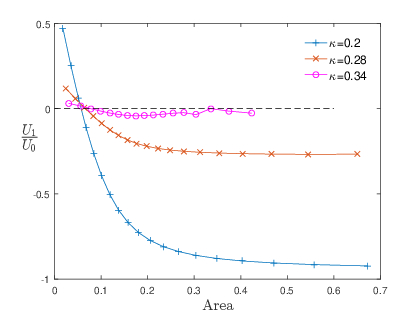
References:
D. G. Crowdy and V. S. Krishnamurthy. The effect of core size on the speed of compressible hollow vortex streets. J. Fluid Mech., 836:797--827, 2018. [ DOI (Open Access) ]
D. G. Crowdy and V. S. Krishnamurthy. Speed of a von Kármán point vortex street in a weakly compressible fluid. Phys. Rev. Fluids, 2(11):114701, 2017. [ DOI | Full Text ]
Point vortex dynamics
Taking a geometrical point of view of three point vortex motion, we consider the evolution of the angles and circumradius of the vortex triangle.
An autonomous system of equations for these variables can be derived.
In the case of finite-time collapse of the vortices, this allows us to obtain formulas linking the Hamiltonian energy of the system with the time of collapse (or expansion).
References:
V. S. Krishnamurthy and M. A. Stremler.
Finite-time Collapse of Three Point Vortices in the Plane.
Regul. Chaotic Dyn., 23(5):530--550, 2018.
[ DOI |
Postprint ]
V. S. Krishnamurthy, H. Aref, and M. A. Stremler.
Evolving geometry of a vortex triangle.
Phys. Rev. Fluids, 3(2):024702, 2018.
[ DOI |
Full Text ]